FPAA-based realization of filters with fractional laplace operators of different orders
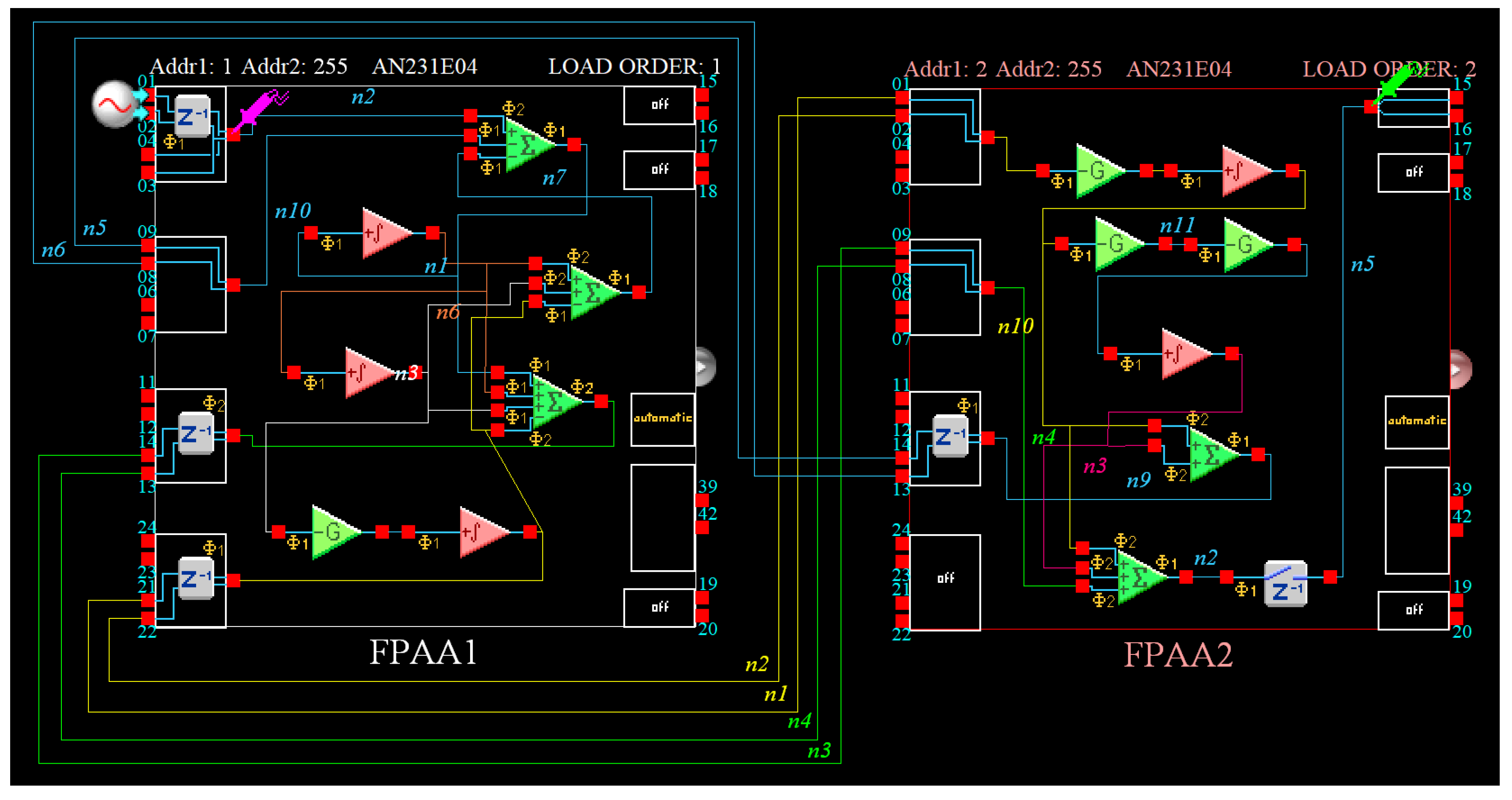
A simple and direct procedure for implementing fractional-order filters with transfer functions that contain Laplace operators of different fractional orders is presented in this work. Based on a general fractional-order transfer function that describes fractional-order low-pass, high-pass, band-pass, band-stop and all-pass filters, the introduced concept deals with the consideration of this function as a whole, with its approximation being performed using a curve-fitting-based technique. Compared to the conventional procedure, where each fractional-order Laplace operator of the transfer function is individually approximated, the main offered benefit is the significant reduction in the order of the resulting rational function. Experimental results, obtained using a field-programmable analog array device, verify the validity of this concept. © 2021 by the authors. Licensee MDPI, Basel, Switzerland.